[SOM] Simple Stress Strain & Elastic Constants [Part-1]

Simple Stress Strain & Elastic Constants [Strength Of Material]

Stress:

Stress is defined as the magnitude of internal resisting force developed or induced at a point against the deformation caused due to load acting on the member.

F=Total internal resistance force developed on the plane of cross-section of the member.

When deformation or strain occur freely in a particular direction then stress developed in that direction will be zero. Because there is no resisting force against the deformation.

Strength:

Strength is defined as the maximum value of stress that a material can withstand without any failure.

From above two equation, the following conclusion can be made:

1)Stress is a second order tensor.

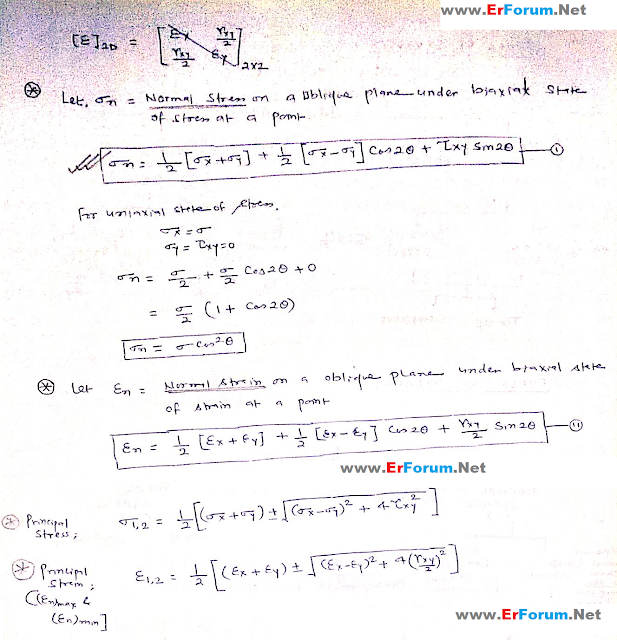
2)Normal stress and Shear stress on any oblique plane passing through a point under uniaxial state of stress can be determined when normal stress on the plane of cross section is known.

3)To determine normal and shear stress on any oblique plane passing through a point under biaxial state of stress, we should know stress developed on two mutual perpendicular plane passing through that point.

Principal Planes:

Principal Planes are complementary oblique plane on which shear stress is zero, but normal stress is either maximum or minimum. Hence these planes are also known as planes of zero shear stress or planes of maximum and minimum normal stress.

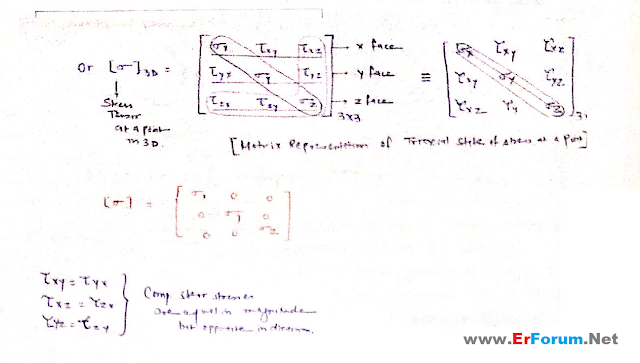
Stress Tensor:

Stress Tensor is used to define the state of stress at a point.

Stress Tensor is a square matrix. That is-

Under triaxial Stress Tensor is 3x3 Matrix

Under biaxial Stress Tensor is 2x2 Matrix

Under uniaxial Stress Tensor is 1x1 Matrix.

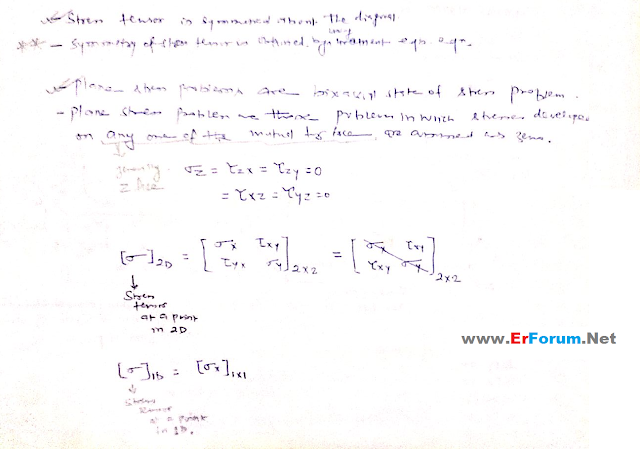
Stress Tensor is symmetrical about the diagonal.

Symmetry of Stress Tensor is obtained by using moment equilibrium equation.

The number of stress components in stress tensor at a point under triaxial state of stress are 9 ( 3 normal stresses and 6 shear stresses).

Number of stress components in a stress tensor to define triaxial state of stress at a point are 6 (3 normal stresses and 3 shear stresses).

The number of stress components in stress tensor at a point under biaxial state of stress are 4. ( 2 normal stresses and 2 shear stresses).

Number of stress components in a stress tensor to define biaxial state of stress at a point are 3 (2 normal stresses and 1 shear stress).

Every Shear stress should be associated with a complimentary shear stress of equal magnitude but in opposite direction.

Strain Tensor:

Strain Tensor is used to define the state of strain at a point.

Strain analysis at a point is similar to stress analysis at that point that is to get strain analysis equations normal stress should be replaced by corresponding normal strain

Total Number of Pages= 9

Download PDF of this Note through below Link:

Simple Stress Strain & Elastic Constants Part-1

Note: If you want to Purchase original Hand Written Notes of this topic [All Parts] . Please write us at ErForum1@gmail.com

Please Like & Share to Support us.